If you need to find the equation of a line passing through the given point and perpendicular to another line, the first thing you need to do is compute the.
Find The Equation Of A Line Perpendicular To Another Line. If you need to find the equation of a line passing through the given point and is perpendicular to another line, the first thing you need to do is compute the slope of the given line. It makes an angle of 90 degrees with a particular point through which the line passes.
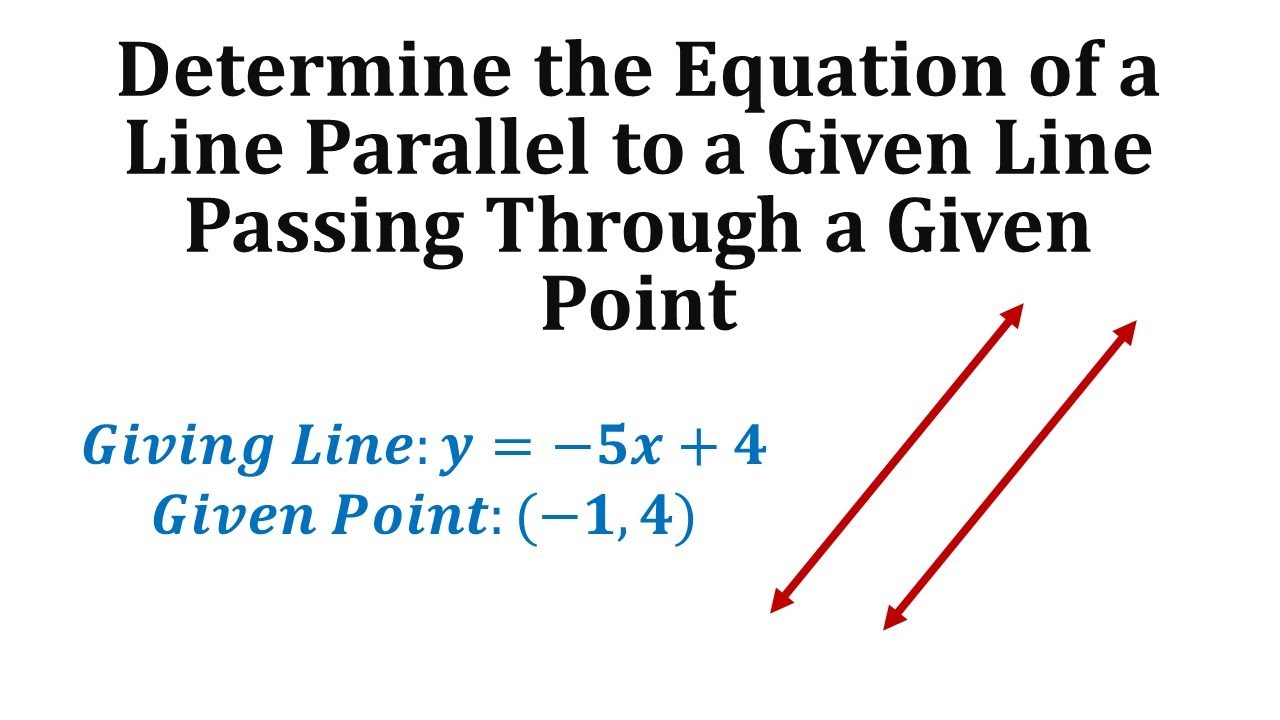
Ex 1 Find the Equation of a Line Parallel to a Given Line From youtube.com
Once we know this, we can use the equation where m is the slope of the line, and is a point on the line. M ′ = − 1 / ( − a b) = b a. To find the equation of a line, we need to know the slope and a point that passes through the line.
Ex 1 Find the Equation of a Line Parallel to a Given Line
Hence the slope of the line perpendicular to line l is given by. Hence, the line can be represented by t as. Obtain the slope of the equation by writing it in the form of y = mx + b. It makes an angle of 90 degrees with a particular point through which the line passes.
Source: brainly.in
Thus, the equation of the line is y = ½ x + 6. Consider the equation of the line is ax + by + c = 0 and coordinates are (x 1, y 1), the slope should be − a/b. Obtain the slope of the equation by writing it in the form of y = mx + b. To find.
Source: youtube.com
In the equation y = mx + c, m is the slope. We discuss how to fi. In general, the normal vector of the plane a ⋅ x + b ⋅ y + c ⋅ z + d = 0 is ( a, b, c). To find the equation of a perpendicular line, first find the gradient of the line.
Source: slideshare.net
M = − a b. Once we know this, we can use the equation where m is the slope of the line, and is a point on the line. Hence, the normal vector of the plane 1 ⋅ x − 5 ⋅ y + 1 ⋅ z − 1 = 0 is ( 1, − 5, 1). Now the product.
Source: courses.lumenlearning.com
We discuss how to fi. Ie 3 x 2 − 3 and at (2,3) the gradient function would be equal to 9 hence for a straight line y = m x + c which is tangent to the curve at (2,3) y = 9 x − 15 since the perpendicular has a gradient which is the negative reciprocal of the.
Source: slideshare.net
This lesson is to help a high school student to understand how to find the line equation if this line is tangent to a circle and perpendicular to another lin. Once we know this, we can use the equation where m is the slope of the line, and is a point on the line. In this calculated equation we observe.
